Calculate Curl Physics
Definition
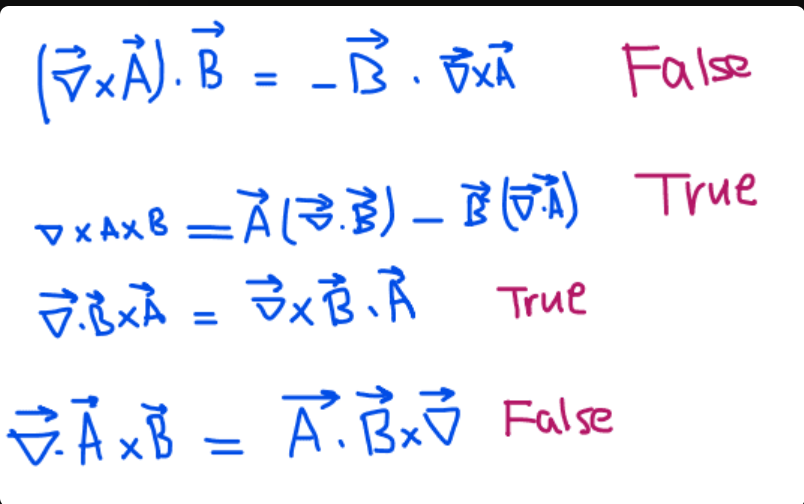
Curl is a vector operator that describes the infinitesimal rotation of a vector field in three-dimensional Euclidean space. It is denoted by ∇ × F or curl F, where F is a vector field.
Characteristics
- Curl is a vector quantity
- It measures the circulation density of a vector field
- The direction of curl is perpendicular to the plane of rotation
- Curl is used in fluid dynamics and electromagnetism
Examples
- Electromagnetic fields: curl of the electric field is related to the time derivative of the magnetic field
- Fluid dynamics: curl of the velocity field represents vorticity
- Weather systems: curl of wind velocity indicates cyclonic or anticyclonic rotation
Non-examples
- Gradient of a scalar field: has no curl
- Irrotational vector fields: curl is zero everywhere
- Conservative vector fields: curl is always zero
Calculation
To calculate curl in Cartesian coordinates:
curl F = ∇ × F = (∂Fz/∂y - ∂Fy/∂z)i + (∂Fx/∂z - ∂Fz/∂x)j + (∂Fy/∂x - ∂Fx/∂y)k
Where F = Fxi + Fyj + Fzk is the vector field, and i, j, k are unit vectors in the x, y, z directions respectively.
Applications
- Analyzing fluid flow and turbulence
- Studying electromagnetic fields
- Investigating atmospheric and oceanic circulation patterns
- Designing efficient propulsion systems
Importance in Physics
Curl is a fundamental concept in vector calculus and has significant applications in various branches of physics. It provides insights into the rotational properties of vector fields, which is crucial for understanding many physical phenomena.
Visualization of curl in a vector field